Review of Brahms and the Shaping of Time, ed. Scott Murphy, University of Rochester Press, 2018

John Paul Ito

View PDF

Suggested Citation

This excellent collection gathers together nine analytically focused essays dealing with temporal aspects of Johannes Brahms’s music. The list of contributors includes many of the living scholars who have contributed most to this area of study, and the title pays tribute to the work of a pioneering forebear, David Epstein.¹ As editor Scott Murphy notes in his introduction, the collection features several kinds of diversity: certainly of approach, but also of genre and of period. (Lied, solo piano music, and chamber music are all well represented, with orchestral music receiving less emphasis and choral music entirely absent; and two chapters focus on the often-overlooked earlier works.) With characteristic wit, Murphy has organized the chapters in what he describes as an aksak rhythm; the 2+2+2+3 scheme begins with pairs of chapters addressing text setting in Lieder, phrase rhythm, and hemiolas, and it concludes with three more tenuously connected chapters under the heading of “Shifting Perspectives.” In this review I shall first offer brief summaries of each chapter, and then reflect on issues of ambiguity and contradiction, amplifying one of the themes present in the text. Some chapters will feature more prominently than others, but this reflects my own interests rather than the quality of the various contributions, which is without exception very high.

1. Overview

1.1.Part One: Setting Texts

The first chapter, by Harald Krebs, applies his concept of the Basic Rhythm of Declamation (BRD) to the music of Brahms. Departing (as does Ryan McClelland) from this collection’s characteristic focus on small numbers of works, Krebs pursues the more general thesis that deviations from the BRD are motivated by text expression, also categorizing Brahms’s uses of BRD-non-conformant text setting.

The BRD combines stress with duration. In traditional German poetry, stressed and unstressed syllables are assumed to fall into mostly regular patterns of feet, with the feet (and the lines and stanzas) having approximately equal duration. A setting is BRD-conformant when the textual stress patterns fit the musical meter and when the durational norms are respected.

After dealing briefly with cases in which there are minor, local disturbances to the BRD (usually lengthened syllables within particularly meaningful words), Krebs turns to his main project, the exploration of more substantial disruptions of the BRD.² He divides these into three main cases. In the first, a rhythmically regular poem is set to varied (BRD-conformant) vocal rhythms, resulting in feet of unequal duration. In the second, a regular poem receives an irregular setting. And in the third, a poem with two-syllable feet (iambs or trochees) is set in triple meter, without using the obvious solution of a 2:1 ratio for stressed and unstressed syllables. In general Krebs’s analyses are extremely convincing. Sometimes the connections with the text are local, dealing with specific words or phrases, and sometimes they are more general, for example foreshadowing a problem that will become apparent only at the end of the poem; but in almost all cases his analyses serve the argument that Brahms’s many deviations from the BRD reflect the poetic content of the texts. The specific reasons Krebs gives for the many metrical deviations of “Wie bist du meine Königin” (34–36) are somewhat weaker, but his discussion of the move to BRD-conformance for the very end of each stanza, connected as it is both to the text and to other musical parameters, is quite persuasive.

In his conclusion, Krebs claims that “Brahms’s declamatory choices can invariably be explained with reference to the text” (43). This immediately follows his observation that Brahms’s text settings are often extreme, either hewing closely to the BRD or else almost abandoning it. But this raises a question about Krebs’s thesis. If the BRD were always Brahms’s default, with all departures motivated by text expression, we might expect something like a bell curve, with the most extreme departures from the BRD occurring quite infrequently. But if the populations at the extremes are in fact the most numerous, then there may be two populations, one in which the BRD is a primary consideration and one in which it is not. Although Krebs argues persuasively for the motivation of departures from the BRD, I find the possibility of an alternate account intriguing.

In Chapter 2, Heather Platt continues the examination of Brahms’s text setting, but with a very different focus: rather than establishing general patterns about Brahms’s irregular text setting, she argues that the specific temporal disruptions in two songs—“O kühler Wald,” op. 72, no. 3, and “Mein Herz ist schwer,” op. 94, no. 3—are unique among Brahms’s Lieder. Platt’s concern is with moments of abrupt discontinuity of pacing, at which the musical action shifts to slower levels of pulse; following Frank Samarotto (1999, ch. 4), she describes these moments in terms of changes in the “temporal plane,” and she associates them with shifts in the level of discourse that lead to shifts in temporality (Hatten 2006, 68), in this case from the present to the past.

In “O kühler Wald,” a sudden temporal discontinuity aligns with an unexpected chromatic turn in the harmony and with an emotionally laden phrase in the text. This is clearly a deliberate expressive effect; Platt’s contribution lies in revealing this moment’s larger implications. A broad perspective is particularly illuminating for “Mein Herz ist schwer,” as the meaning of the pivotal moment is less transparent. Platt draws on a concept of romantic melancholy to frame a close examination of several moments in which disruption of pacing interacts with harmonic design, form, and textual meaning. Platt’s analyses are models of thoroughness, not only drawing intraopus connections but also summoning relevant passages from other Lieder to dispel potential competing interpretations. This comparative approach becomes central in the final section, as she considers but then rejects passages that might seem to contain similar effects, supporting her argument that these two Lieder stand alone.

1.2.Part Two: Measuring Phrases

In Chapter 3, Jan Miyake examines “Gestillte Sehnsucht,” op. 91, no. 1, for alto, viola, and piano, which offers a rich variety of phrase expansions. An early example appears at the end of the introduction, where a four-bar hypermeasure takes five notated measures because of a passage in which the heard meter expands from the notated $$2\atop4$$ to $$6\atop8$$. Immediately following this, an unusual phrase length arises in a very different manner, as the first four measures of the introduction are expanded by adding anacrustic measures in the voice that form an integral part of the vocal melody. Beyond remarking on local effects, Miyake makes a strong case for twelve-bar units as basic building blocks in the song’s construction; these twelve-bar units are often expanded (and occasionally contracted) in ways that intensify the song’s overall theme of longing. The song thus offers an unusually rich demonstration of the expressive possibilities of phrase rhythm, between the many ways in which phrase length is manipulated and Brahms’s exploitation of different possible placements for the triple hypermetrical layer within the overall twelve-bar design.

In the second chapter on phrase rhythm, Samuel Ng examines the question of five-bar units in Brahms, focusing on three late piano pieces in which they are particularly prevalent.³ His central point—supported by quotes from François-Joseph Fétis and Anton Reicha—is that five-bar phrases should have integrity that does not derive from four-bar models. His goal is then to demonstrate the necessity of the five-bar units. This is not a matter of showing that four-bar derivations are impossible, as each of his discussions includes credible four-bar models. Rather, Ng argues that the five-bar themes cannot be reduced to four-bar models without losing essential structural and expressive features. The five-bar constructions—and especially, in one case, those measures that might have been understood as insertions—have important consequences for later development, consequences that encompass phrase rhythm, form, and expression.

In the Ballade, op. 118, no. 3, the third measure of the initial theme could potentially be understood as an insertion, but Ng shows that it drives much of the piece’s drama. Because of this measure, the theme surges forward in the middle only to pull back before the cadence; Ng relates many key moments to this, hearing the movement as a tragic failure of will whose seeds are contained in the first five measures. The analysis of the Intermezzo, op. 117, no. 3, is similar, as a proposed four-bar model is shown to lack two crucial features of the actual theme: an aspiring upward motion to the subtonic, and then a downward collapse through a diminished octave to the leading tone. Max Kalbeck, taking a cue from the epigraph to op. 117, no. 1, has suggested a hidden text for this Intermezzo (1914, 280), and Ng draws connections between this text and various versions of the theme to reveal an overall narrative about the death of hope. Ng’s concluding analysis, of the Rhapsody, op. 119, no. 4, contains the most stunning analytical denouement of the book; beyond discussing one of its subcomponents below in the general discussion, I shall not preview it here in order to avoid spoilers. I urge readers to experience it for themselves.⁴

1.3.Part Three: Recasting Hemiolas

Given my own interests, the chapter that I have found the most impactful, and that has had the furthest-reaching implications, has been McClelland’s on the stabilizing role of hemiolas. Expanding significantly on a remark in one of Channan Willner’s articles, McClelland observes that hemiola, although considered a metrical dissonance, often serves to clarify and stabilize the notated meter in Brahms’s music.⁵ After a brief general exposition of the issues involved, the chapter looks at cases in which hemiola is used to return to the notated meter following passages in which an alternate heard meter prevails; McClelland asserts that this occurs most frequently with half-measure displacements in $$6\atop8$$, but he also considers other cases, such as a quarter-note displacement in $$3\atop4$$.⁶

The chapter next takes up cases in which the stabilizing role of hemiola operates at a hypermetrical level, sometimes returning to a previous phase alignment of strong measures and sometimes asserting a new one. These cases can also involve metrical dissonance within the measure; a particularly convincing instance is given in Examples 5.14 and 5.15, in which McClelland shows through recomposition that hemiola resolves a metrical dissonance much more effectively than a straightforward reassertion of metrical consonance. In a final section, the chapter takes an exploratory turn, trying to hear hemiolas that do not appear to play a stabilizing role as if they did. He offers three very satisfying analyses, one of which resonates strongly with Edward Klorman’s recent work (2016) in suggesting that different players within a chamber ensemble might hear and project hypermeter differently.

In the second chapter on hemiola, Richard Cohn gives close attention to several passages from the first movement of the Piano Sonata in F minor, op. 5, arguing that the quality of the works of the 1850s is unjustly demeaned by the use of the term ‘first maturity’ for the works of the 1860s.⁷ Cohn lists five characteristics of the movement that, in addition to being hallmarks of Brahms’s later style, also involve “ordering, pacing, and large-scale planning” (179), stressing that these are not merely superficial badges of compositional maturity that could be pinned onto otherwise immature works. In this chapter he focuses on two of these characteristics: the sophisticated use of hemiola, and a deep engagement with ambiguity.

The hemiolas considered by Cohn appear primarily as hemiolic cycles, a construction first explored by Ng (2006). In a regular hemiola, two 3-beat metric spans are replaced by three 2-beat spans. In a hemiolic cycle, one or more (displaced) 3-beat spans are interpolated between 2-beat spans of the hemiola. In contrast to Ng’s emphasis on the patterning of the musical surface, Cohn digs deeply into the conception of the 3-beat spans as interpolations, appealing to Heinrich Christoph Koch’s concept of the parenthesis. As a result, his primary evidence is not the salience of the hemiolic cycle but rather the coherence and plausibility of the underlying, unexpanded hemiola as material for a compositional process.

This is an important move, as his readings in terms of hemiolic cycles are not particularly promising on first glance. To take the most obvious example, his hearing of the opening measures must overcome both the thundering low-register octaves on the downbeats and the chord change on the downbeat of m. 2. (Example 1 reproduces Example 6.2a. Cohn claims there are no harmony changes into mm. 2–4, where I hear a variant on the idiomatic move from ii^ø$$4\atop2$$ to V$$6\atop5$$ across the first barline.) I find the hemiolic cycle only tenuously supported in the first appearances of the passages Cohn examines. But Cohn then proceeds to trace an astonishingly consistent web of connections between those readings and other appearances of the material throughout the movement. Cohn’s chapter thus offers both an extremely interesting analysis and a case study in methodology, and I expect readers to have varied responses to its rather skewed balance between ready connection with aural experience and compelling intellectual argument.

1.4. Part Four: Shifting Perspectives

Opening the final set of three less obviously connected essays, Samarotto’s chapter on the Piano Sonata in F$$\sharp$$ minor, op. 2, makes the volume’s most novel theoretical advance. Focusing mainly on the first movement, Samarotto uses the Sonata as a case study in the utility of two overarching metaphors: container and wave. Inspired partially by Jérôme-Joseph de Momigny, the container represents aspects of music that delimit and define, while the wave represents musical motion (even “pure motion,” 217), animating containers but also striving against them. “Without motion, there is no music; without boundaries, that motion is meaningless” (210). For its introduction both to the main ideas and to Samarotto’s vivid prose, the first paragraph is worth quoting in full.

Brahms’s Piano Sonata in F-sharp Minor—his opus 2, but composed before opus 1—begins with a splash (see ex. 7.1). A splattering arpeggio ripples up the keyboard; its force (Brahms marks it energico) rebounds into rising melodic octaves whose surge is abruptly halted, as if hitting a wall. Another splash and another surge brings another lurching slap against that wall—and this time an even greater swell rises up, slowly gathering force, hitting its registral peak and cascading downward to crash against the rock of the opening arpeggio once more. (207)

Samarotto helpfully clarifies that “containment, whether of temporal units, tonal spaces or formal plans, is grounded in actual sounding events; wave is a listener’s imagination of motion filling, transcending, or overflowing those events” (209). The focus on imagined motion is key: Samarotto is essentially offering a new approach to musical narrative, a way of conceptualizing how a piece goes and how its events are meaningful.⁸ The bulk of the chapter consists of a detailed examination of the Sonata’s first movement, highlighting the interplay of local detail with larger pitch organization, and often employing reductional analyses (lightly and not-so-lightly Schenkerian). Samarotto presents the movement as a pitched conflict between container and wave, a conflict waged across many parameters and levels of scale, and he offers a compelling account. The remaining movements of the Sonata are treated more briefly, in sketches that show persuasively that container and wave continue their dynamic interplay through the rest of the Sonata. In his concluding paragraph, Samarotto indicates that future work on Brahms’s use of container and wave will show him choreographing more precise and delicate pas de deux between these protagonists, and that it will allow us to more fully appreciate Brahms’s subtlety in shaping time. In this chapter, Samarotto gives every indication that he will be able to realize those aims.⁹

Eytan Agmon’s 1991 essay, “Rhythmic Displacement in the Fugue of Brahms’s Handel Variations,” is included because it is (still, as of the writing of this review) not easily accessible via online archives. The chapter’s focus is metrical displacement. Using compound meter in the original sense, many eighteenth-century fugues in common time feature frequent incidental half-note displacements of the subject; Brahms’s subject strongly implies first quarter-note and then eighth-note displacements of the heard downbeats, and he plays with these implications throughout the fugue. This play has two particularly interesting features: (1) the “rightness” of the notated meter is grounded by its relationship to Handel’s theme rather than by perceptual clarity; and (2) in the body of Brahms’s fugue, the play never involves actual displacement of the subject, but rather a variety of adjustments that nudge perception in one direction or another—the shifts are always in the ear, never on paper as in the original eighteenth-century practice. In the concluding measures, however, Brahms does shift the head motive both by a quarter note and by an eighth note, a sort of final acknowledgement of the games he has been playing.¹⁰ This chapter stands out in the volume for its somewhat fleeter pacing, presenting insightful analysis as a quick and easy read.

The final chapter, Murphy’s account of what he calls “durational enharmonicism” in the opening of the Double Concerto, op. 102, weaves together an impressive variety of strands. Most centrally, Murphy develops pitch-time correspondences that trace back through his own earlier work (2009) to an influential article by David Lewin (1981), but he also considers listener perception, performance practice, and biography.¹¹

Durational enharmonicism refers to the potential of a performed duration to receive either of two metrical interpretations. It can arise in the beginning of the cello’s opening cadenza through a confluence of factors: the initial rhythm of triplet half notes is both rare and difficult to project clearly; the monophonic context provides no other cues to meter; the cadenza should be played in the style of a recitative, but in tempo, with the second instruction rarely heeded; and, at least in some recordings, the performed durations lie between metronomic dotted quarters and triplet halves, so that listeners without scores could have real uncertainty about which they were hearing.¹² After arguing for the viability of hearing the cadenza start with dotted quarter notes, Murphy makes an elegant case for the actual triplet half notes as more likely. The chapter shows how an alternate hearing of the start of the cadenza might play out in what follows, and it gives an experiential account of the (many) potential paths that durational enharmonicism can open up. It concludes with some intriguing connections with the personal history behind the Concerto, suggesting that the cadenza’s metrical complexities may be an unflattering portrait of its dedicatee, Joseph Joachim.

This is an impressive chapter, but it leaves me ambivalent about just how much its multifaceted virtuosity has accomplished. If the main point is analysis, the amount of new technology developed is formidable, especially as few performances of the in-tempo recitative allow durational enharmonicism to come into play (277–278).¹³ And if the main point is the theoretical advance, I wonder how many pieces it will illuminate, especially as the crucial ambiguity depends on the monophonic context (274). The historical and biographical connections seem therefore crucial, as they counterbalance these concerns, allowing the analysis to speak to issues that go beyond the piece itself.¹⁴

2. Commentary

The essays in this volume share many strengths, and I trust they have already been made clear. Here I briefly address two criticisms, and I reflect on the issue of ambiguity—a theme which is certainly prominent, but which could have emerged with even greater salience.

One weakness is that a number of the chapters offer an overabundance of analytical detail. I admire their thoroughness and insightfulness, but in several places a more streamlined argument would have been more effective, certainly more efficient.¹⁵ A second weakness is that the book lacks sustained, explicit engagement with more recent perspectives on such things as unity, ambiguity, and the nature of analysis (where “more recent” refers to the last thirty years or so). This charge cannot be laid at the feet of any one chapter; rather, addressing this issue would probably have required commissioning at least one additional essay. As examples of perspectives that are well represented here, both Krebs and Platt frame their chapters as responses to critiques of Brahms’s text setting, in some passages taking a rather defensive tone; their goal is to rehabilitate his reputation(!). Cohn also explicitly strives to defend some of Brahms’s works, and like Krebs and Platt he sets out to do this by means of analysis.¹⁶ In a similar vein, Ng concludes his chapter with themes of determinism and necessity, writing, “strange as these phrases . . . may be, we could still find ourselves agreeing with Clara Schumann’s simple and yet profound statement, ‘es muß so sein’” (134). I do not intend to pull this fine book into the often acrimonious debates that have surrounded these questions, nor do I have any interest in taking sides myself, especially as these chapters all have meaning and value that would allow them to be reembedded in different frames by readers so inclined. But it is worth noting that, despite the many forms of diversity that are so admirably present in this volume, one kind of voice is conspicuous by its absence. To take one obvious example, this book was published twenty-five years after Kevin Korsyn’s review (1993) of the volume that stands as its most direct predecessor, George Bozarth’s edited collection Brahms Studies: Analytical and Historical Perspectives (1990); surely an author could have been found to engage explicitly with the ideological and metatheoretical issues Korsyn raises.

The issue of ambiguity arises frequently in analyses of Brahms’s music, and it receives extensive attention in this volume, especially in the chapters by McClelland, Cohn, and Murphy. When examining time in Brahms’s music, however, ambiguities can be so saliently hearable that it is worth underscoring their nature even more explicitly. I shall therefore amplify four foundational points regarding temporal ambiguity in Brahms raised by this volume. First, ambiguity comes in two basic forms: sometimes a given instance is ambiguous in that it can be interpreted in multiple ways (as in Murphy’s construals of durational relationships), and sometimes the underlying material is ambiguous, with individual instances of this material unambiguous in and of themselves but different from each other (as in McClelland’s analysis of hypermeter in the second theme of the first movement of the Piano Trio, op. 101 [pp. 171–173]). Second, in relation to both basic forms, Brahms is extremely adept at subtly manipulating accentuation and weight in order to adjust the likelihood of one interpretation versus another. (Cohn emphasizes this point, especially in Table 6.1 [190].) Third, the relatively unambiguous—but different—interpretations of various instances of the same material can create paradoxes and deep contradictions, as seen in that same op. 101 analysis. Finally, divergent interpretations of a single instance can lead to extremely salient perceptual contrasts, as Murphy highlights in his discussion via analogies with visual illusions such as the rabbit-duck. In what follows I explore the final two points, in reverse order.

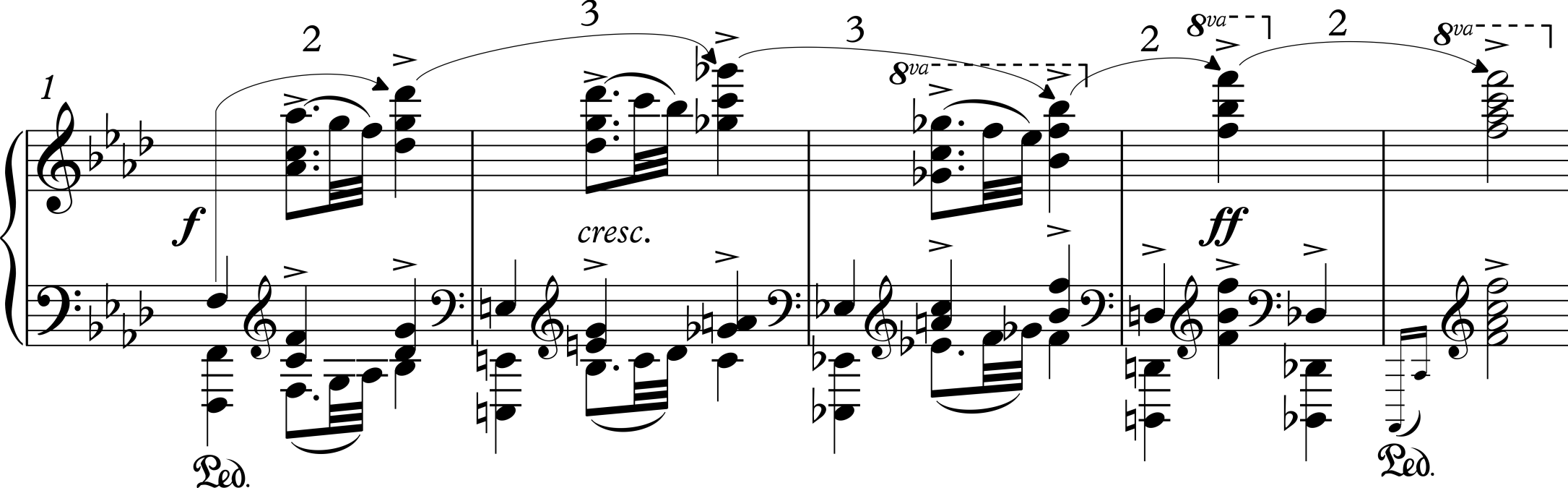
One of the clearest examples in the book of how analysis might destabilize simplistic ontologies of the musical work—and thus respond to the sorts of issues raised by Korsyn (1993)—is Murphy’s claim that there are thirty-two different ways in which a listener might understand meter in the opening cadenza from op. 102. (Murphy does not discuss this implication of his analysis at length.) Certainly it is a prime example of true perceptual ambiguity, of the music changing its nature under a different hearing. But this is a very unusual case; for a simpler one we can return to Ng and to the opening five-bar phrases of the Rhapsody, op. 119, no. 4. (Example 2 reproduces the first system from Ng’s Example 4.12.) Citing remarks in liner notes by pianist Richard Goode, Ng observes that the final sets of three $$2\atop4$$ measures in each of the initial phrases can be heard instead as two measures in $$3\atop4$$.¹⁷ But Ng does not mention that the same music can also be heard without departures from the notated meter. Because of this, the theme can take on two quite different characters. As notated, the theme seems aggressively obstinate, due especially to the weak-beat cadence in m. 4. Because the cadential harmony is repeated during the following measure, the harmonic rhythm forms a less-preferred short-long pattern, which, as Fred Lerdahl and Ray Jackendoff point out (1983; 80, 84–85), often relies on phenomenal stress. Especially as it begins a cadence, the downbeat of m. 4 is likely both to acquire strong accentuation and to be heard as hypermetrically strong. Where the notated meter insistently pounds out “too many” downbeats, the 2+2+3+3 meter of the alternate hearing is smoother in quite an unusual way that combines the regularity of four-bar hypermeter with the irregularity of the shifting measure size. Note that the downbeat of m. 4, hypermetrically strong if heard in the notated meter, is not accented either metrically or otherwise in this alternate hearing. To see how carefully Brahms has calibrated the balance between the two potential hearings, compare a more obvious instance of the same alternate metrical and hypermetrical hearing, the opening five measures of the final movement of the Piano Trio, op. 87. In the Trio, the alternate hearing is strongly supported by (if not created by) parallel material. In the Rhapsody, by contrast, the strongly parallel mm. 2 and 3 are cleft by the change of heard meter: though the material can still be described as falling on beats 1 and 2, there are perceptual consequences of placing those beats in a metrical frame that contains three beats instead of two.¹⁸ In sum, the two hearings of op. 119, no. 4, present two very different ways in which we can experience the same music. It is as if Brahms has used the same marks on the page to write two quite different pieces, only one of which we can hear at a time.

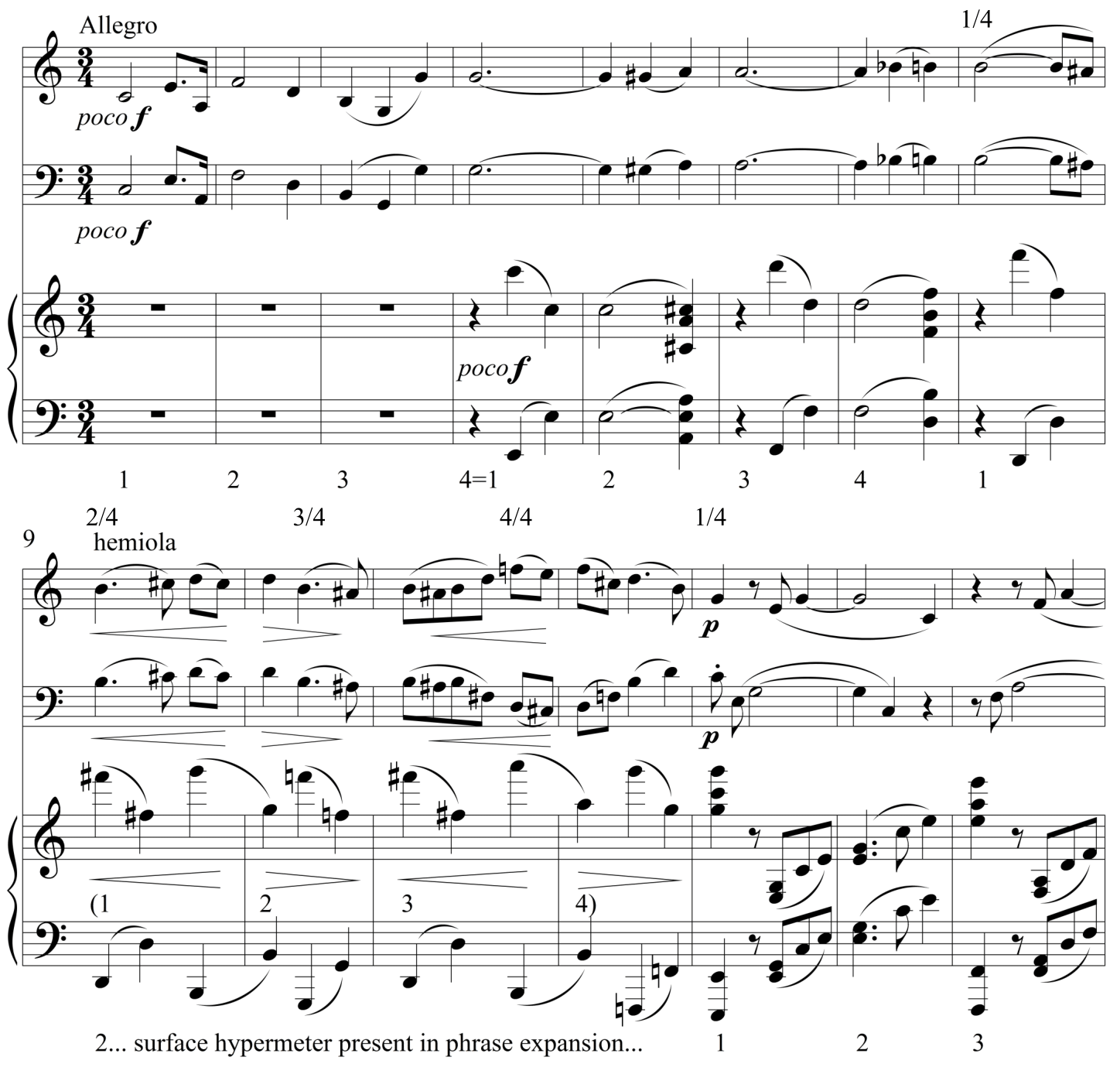
In turning now to paradox and contradiction created by ambiguity, two passages examined by McClelland will serve as helpful examples of cases in which divergent analytical clues lead to irreconcilable conflicts. Example 3 shows his Example 5.13, the opening of the Piano Trio, op. 87, together with some extra analytical annotations that I have added. McClelland’s analysis, responding especially to the piano part, has the surface hypermeter align with the hemiola in mm. 9–12 (note the parallel in content between hyperbeats 1 and 2 [mm. 9–10] and hyperbeats 3 and 4 [mm. 11–12]). Shifting attention elsewhere would generate a different result. The violin melody that starts in m. 8 contains a strong parallel: B–A$$\sharp$$–B–C$$\sharp$$–D–C$$\sharp$$–D is answered by B–A$$\sharp$$–B–(A$$\sharp$$–B)–D–F–E–F. McClelland’s hearing obscures this relationship, as the first statement begins on a hypermetrical downbeat and the second in the middle of a hypermetrically weak measure, given weight only as the third beat within a hemiola that does not disrupt the heard meter (other than by asserting its beginning as hypermetrically strong). If, however, we hear not a hemiola but a double hemiola, and if this leads us into a heard common-time meter, then the two parallel melodic statements will begin on the downbeats of the first and third measures of a four-bar hypermeasure; this hearing is indicated by the additions to McClelland’s example, the hyperbeats shown above the staff using my fractional notation (Ito 2013).¹⁹ I can mainly follow the piano, as McClelland does, or I can mainly follow the strings, as in my alternate analysis, but I cannot find a hearing that synthesizes the two.

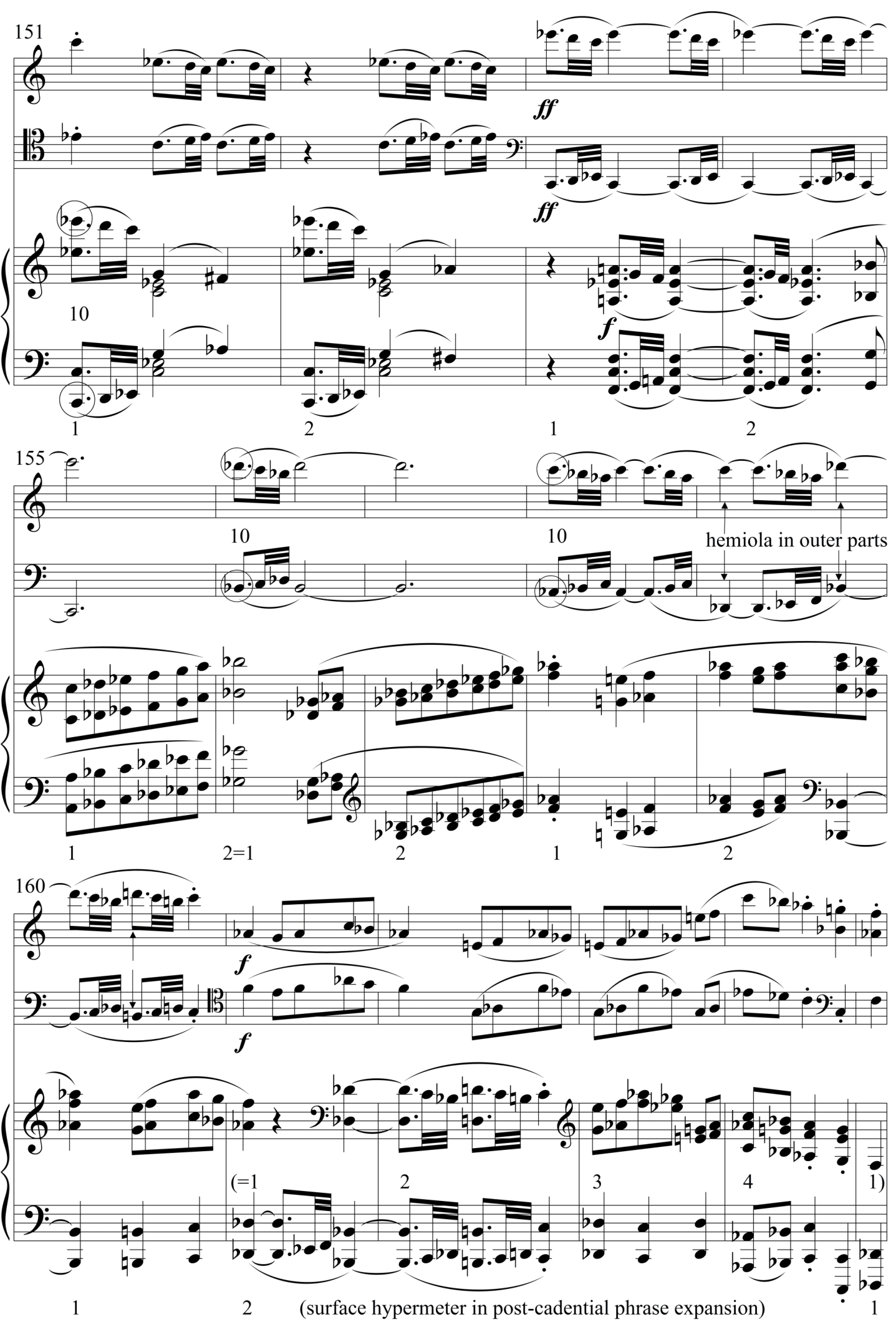
The tensions are even more acute in the final passage that McClelland discusses from the first movement. Example 4 shows his Example 5.16. McClelland uses vertical arrows between the string parts to draw attention to a hemiola in mm. 159–160. According to McClelland, this hemiola has two functions, preparing both for the reemergence of metrical and hypermetrical clarity in mm. 161–164, and for the adjustment of hypermetrically strong and weak measures in m. 161. The story of this hemiola is more complex, however. It first appears in mm. 153–154, primarily in the strings. The figure is quite syncopated: after its beginning, ties across the hemiola’s strong beats prevent them from receiving accentuation, while the hemiola’s weak beats receive attacks that begin long notes. The strings are clearly the leading voice in these measures, given that their figure starts on the downbeat, parallels the preceding two measures, and is paralleled by mm. 156 and 158. The piano echoes the strings in a rhythmic canon at the quarter note. The canonic treatment of the syncopation creates a metrical double negative—the piano’s echoes of the strings’ syncopated attacks fall on strong beats of the hemiola.

In mm. 153–154, the piano’s metrically aligned version of the hemiola clearly has a subordinate status. But when the piano’s version returns in m. 161, it is no longer a double negative: now it begins on the downbeat, and rather than being an echo it is the only version of this material. We can then see mm. 158–160 as a metrical transition (Temperley 2008). When this material begins in m. 158, the long notes should, in parallel with mm. 153–154, be heard as syncopated. (Under this hearing, McClelland’s hemiola arrows in m. 159 mark weak beats.) But because this material continues for three measures instead of two, by its end McClelland’s interpretation makes the most sense. This lets the hemiola end with a complete unit, transitioning neatly into the non-syncopated version of the hemiola material in mm. 161–162. As in McClelland’s discussion of the second theme from the first movement of op. 101, we have a predicament: the basic principle of parallel material receiving a parallel analysis, which has been invoked so often in this book, comes under direct threat. But unlike the passage from op. 101, which has two reasonable moments of adjustment, this passage offers no clear solutions to the listener seeking a coherent path through this metrical muddle (or at least I have not found any). Not just as an intellectual puzzle but as a perceptual experience, this is a passage that, by juxtaposing two irreconcilable accounts of order, insists on a disorienting descent into chaos. It is a good example of Korsyn’s observation that many of Brahms’s works cannot be apprehended as single grand objects, and thus that he “prevents us from identifying unambiguously with the work, from accepting it as an apparently natural event” (1993, 98). This passage numbers among the thornier examples of metrical difficulties in Brahms, but it does not approach standing alone. Some of these difficult passages can be cracked open to reveal neat solutions, others cannot.

Given how thoroughly the broader literature on Brahms has explored questions of ambiguity and paradox, including in studies related to time, I do not fault the book—and certainly not any of the individual chapters—for not highlighting these issues more comprehensively. But as this volume can now deservedly stake a claim to being the go-to resource for time in Brahms, these are issues worth emphasizing, especially for readers who may come with less background.

Even more worthy of emphasis, however, is the value of the contribution made by this book. Brahms and the Shaping of Time will be extremely helpful for scholars working on Brahms or on time in music, and for scholars working on both it will be indispensable. The back cover endorsement by Frank Heidlberger calls it a must-read for performers, and that accolade is fully earned in terms of content (though it may require an asterisk on account of the quite scholarly writing).²⁰ The book could certainly serve effectively as the backbone of a class on time in Brahms, especially given the diversity of repertoires and approaches; students would come away with an excellent overview. In all, with its depth of analytical exploration, its sensitive musicality, its rich interconnections with other literature, and the clarity and often the elegance of its writing, this is a book that will amply reward its readers.

---

John Paul Ito is Associate Professor of Music Theory in the School of Music at Carnegie Mellon University. He received an SB in music from MIT, an MM in viola performance from Boston University, and a PhD in music theory from Columbia University. His main areas of research are meter and hypermeter and their connections with cognition, performance, and the history of musical style. He has published in Bonner Beethoven-Studien, The Journal of Musicology, The Journal of Music Theory, and Music Perception, and his book Focal Impulse Theory: Musical Expression, Meter, and the Body will be published in 2020 by Indiana University Press in the series Musical Meaning and Interpretation.

References

Agmon, Eytan. 1991. “Rhythmic Displacement in the Fugue of Brahms’s Handel Variations: The Refashioning of a Traditional Device.” Studies in Music from the University of Western Ontario 13: 1–20.

Bozarth, George S., ed. 1990. Brahms Studies: Analytical and Historical Perspectives. Oxford: Clarendon.

———. 2000. “Brahms, Johannes.” In The New Grove Dictionary of Music and Musicians, 2^nd ed., ed. Stanley Sadie, 4: 183. London: MacMillan.

Cox, Arnie. 2016. Music and Embodied Cognition: Listening, Moving, Feeling, and Thinking. Bloomington: Indiana University Press.

Epstein, David. 1995. Shaping Time: Music, the Brain, and Performance. New York: Schirmer.

Hatten, Robert S. 2006. “The Troping of Temporality in Music.” In Approaches to Meaning in Music, ed. Byron Almén and Edward Pearsall, 62–75. Bloomington: Indiana University Press.

Ito, John Paul. 2013. “Hypermetrical Schemas, Metrical Orientation, and Cognitive-Linguistic Paradigms.” Journal of Music Theory 57: 47–85.

———. 2018. “Brahms and the 1.5-Length Bar.” Paper presented at the annual meeting of the Society for Music Theory, San Antonio, TX.

———. Forthcoming. Focal Impulse Theory: Musical Expression, Meter, and the Body. Bloomington: Indiana University Press.

Kalbeck, Max. 1914. Johannes Brahms. Berlin: Deutsche Brahms Gesellschaft.

Klorman, Edward. 2016. Mozart’s Music of Friends: Social Interplay in the Chamber Works. Cambridge: Cambridge University Press.

Korsyn, Kevin. 1993. “Brahms Research and Aesthetic Ideology.” Music Analysis 12: 89–103.

Larson, Steve. 2012. Musical Forces: Motion, Metaphor, and Meaning in Music. Bloomington: Indiana University Press.

Lerdahl, Fred, and Ray Jackendoff. 1983. A Generative Theory of Tonal Music. Cambridge: MIT Press.

Lewin, David. 1981. “On Harmony and Meter in Brahms’s op. 76, no. 8.” 19th-Century Music 4: 261–265.

Murphy, Scott. 2009. “Metric Cubes in Some Music of Brahms.” Journal of Music Theory 53: 1–56.

Ng, Samuel. 2006. “The Hemiolic Cycle and Metric Dissonance in the First Movement of Brahms’s Cello Sonata in F Major, op. 99.” Theory and Practice 31: 65–95.

Rink, John. 2002. “Analysis and (or?) Performance.” In Musical Performance: A Guide to Understanding, ed. John Rink, 35–58. Cambridge: Cambridge University Press.

Samarotto, Frank. 1999. “A Theory of Temporal Plasticity in Tonal Music: An Extension of the Schenkerian Approach to Rhythm with Special Reference to Beethoven’s Late Music.” PhD diss., Graduate Center of the City University of New York.

Temperley, David. 2008 “Hypermetrical Transitions.” Music Theory Spectrum 30: 305–325.

Webster, James. 1979. “Schubert’s Sonata Form and Brahms’s First Maturity (II).” 19^th-Century Music 3: 52–71.

1. Through a curious oversight, no mention is made in the Introduction to Epstein’s Shaping Time (1995).
2. The disruption of the BRD warrants a term and thereby an acronym; based on Krebs’s word choices, Disrupted Declamational Rhythm and Distorted Declamational Rhythm would be obvious candidates.
3. I have two small quibbles with Ng regarding five-bar units in Brahms. First, he states that “while abundant examples of irregular phrase lengths can be found in his earlier works, nowhere else does Brahms seem as engrossed in the potential of five-measure phrases as in these late piano pieces” (111). But the Variations on a Theme by Joseph Haydn, op. 56, also explore the possibilities of five-bar construction, showing many derivations of five-bar units. And if only prevalence is required, then we should also consider op. 95, no. 3, and arguably also op. 21, no. 1, which alternates four- and five-bar units. Second, although Ng is admirably cautious about connecting five-bar organization with the Hungarian flavor detected by Clara Schumann in op. 119, no. 4, I would list the final animato from the fourth movement of the Quintet, op. 111, the Zigeunerlieder, op. 112, nos. 4 and 5, and the middle section of the slow movement from the Clarinet Quintet, op. 115, as passages combining gypsy associations with five-bar construction, adding also op. 118, no. 3, one of the other pieces Ng considers.
4. This chapter unfortunately includes most of the production flaws found in the book. Readers should expect a few miscalled figures and examples, and also the detritus of an overly hasty conversion from Acoustical Society of America (ASA) to Helmholtz pitch notation. The most serious potential confusion arises in Example 4.11: case (1)’s tonal diminution compresses its final two measures into one, the final case should be labeled as (5), and its diminution’s last two measures should match those of case (2) and those of a correctly rendered case (1), not those of the actual piece. This is perhaps a good place to acknowledge Murphy’s generous sharing of a late draft of the entire book with me, which I used to confirm my observations about the incorrect rendering of Example 4.11.
5. He also suggests that when hemiolas perform a stabilizing function, they are not really metrical dissonances at all (160, 174n1); though I disagree, this provocative idea is worth further discussion. Regarding the empirical implications of McClelland’s thesis, I have performed a corpus study of metrical dissonance in Brahms’s works with opus number, and while instances of simple hemiola were too numerous to include, I have tabulated instances of potential changes in heard meter. As expected on the basis of McClelland’s thesis, hemiola within an alternate heard meter is quite rare. (I will make a fuller assessment of McClelland’s claims when I publish the full results.) One such case, the one that most clearly functions as an exception that proves the rule, is found in the first movement of the Third Symphony. In m. 50, Brahms begins to give accentuation to the third and sixth quarter-note beats of the $$6\atop4$$ meter. As often happens when introducing metrical shifts, he gradually increases the emphasis on these beats, so that a listener becomes more and more likely to shift the heard downbeat, but without a single, defined point at which this is most likely to occur. At the end of m. 54, Brahms introduces a strong hemiola pattern that lasts two measures; unless extreme efforts are made, this makes the shifted downbeats certain to be heard. Based on McClelland’s work, it seems very likely that this occurs in part because hemiolas have such a strong orienting function. Ironically, this is followed directly by a very clear reassertion of the notated downbeat.
6. Within this section, Example 5.5 is replaced by something from a different book entirely; fortunately, the reader who looks up the relevant measures in the score will be able to supply the few annotations on the missing example without difficulty based on the text.
7. Cohn briefly sketches the history of this designation, from its coinage by Donald Francis Tovey (Webster 1979, 53n2) to its inclusion in the New Grove Dictionary (Bozarth 2000).
8. As a result, there are broad kinships of intention, if not content, with the work of Steve Larson (2012) and Arnie Cox (2016). More directly, Samarotto’s metaphors could be used in the kind of performer’s analysis proposed by John Rink (2002), analysis that focuses on shape more than on structure.
9. Noting one small error in Example 7.5a will make the text easier to follow: the final note in the actual melody should be B, not C$$\sharp$$.
10. Agmon offers a less glib reading of this moment.
11. Both earlier articles focus on music by Brahms.
12. Following a number of uncited precedents, Murphy uses a window of ten percent on either side as a generous estimation of a just-noticeable difference. Performance is indeed crucial in opening or closing the options Murphy discusses; e.g., there are few recordings in which the orchestra’s second chord could be construed as stronger metrically than its third (Ex. 9.3, 271).
13. Murphy urges the reader to try out the proposed hearings with a sempre in tempo performance (287), but he does not indicate whether any of the performances he surveys come close enough to serve. The most obvious solution is to use notation software; perhaps Murphy could post the relevant source files publicly, e.g. on his blog. But even here adjustments are needed, as sempre in tempo performances of these 9:8 or 8:9 tempo relationships just fail his “generous” (266) ten-percent standard. For all thirty-two possible paths through the cadenza to be hearable in one performance, one would need a recording in which each juncture’s durations split the difference between dotted and triplet interpretations.
14. In Figure 9.2b, the number 0.9 should not be crossed out, and the upper lines of p. 287 twice call figures using the wrong numbers.
15. Some of the responsibility for this belongs to Murphy; beyond general editorial responsibility, several passages credit him with analytical points that were perceptive but not essential.
16. Samarotto’s essay is a noteworthy exception to this pattern, as he suggests in his conclusion that he does not actually view the op. 2 Sonata as being entirely successful, despite the compelling patterns that his analysis reveals. Samarotto’s viewpoint could be used to reframe Cohn’s argument; for example, perhaps Cohn has shown not that the works of the 1850s are mature, but rather that any charge of immaturity cannot rest on analytical criteria.
17. Ng uses the term ‘reverse hemiola’ for these measures; this term makes sense (only) because the measures that are one-and-a-half times the length of the prevailing measures appear in pairs in this passage. Such measures occur frequently in Brahms, and because they do not always come in pairs, I prefer the term “1.5-length bar” (Ito 2018). They can also be heard in the slow movement of the Fourth Symphony, mm. 84–86 (Example 5.8, 152–153).
18. As a further indication of the balance between the two hearings, there are plenty of signs in Goode’s recording that he heard the alternate meter but no barriers to hearing the notated meter. I argue elsewhere that when alternate heard meters are strongly projected in the score, it often takes special efforts on the part of the performer to make the notated meter a viable option for a listener (Ito forthcoming)—and Goode was presumably not making any such attempts.
19. Though the parallel at the start of the figure is clearer in my analysis, McClelland’s is more consistent in its treatment of the final three notes of the figure, D–C$$\sharp$$–D / F–E–F. Like Miyake’s hearing of op. 91, no. 1, and Ng’s hearing of op. 119, no. 4, my analysis results in a unit of five notated measures that is heard as a four-bar hypermeasure because of an alternate heard meter. It also agrees with McClelland’s analysis in making mm. 8 and 13 into hypermetrical downbeats, but without the disjunction between underlying and surface hypermeters.
20. I dream of a duo with the comedic gifts and enthusiasm of Brett Yang and Eddie Chen of TwoSet Violin who might popularize performance-oriented theory and analysis on YouTube. Any volunteers?